triangles

Definition Of Triangles 

triangle

ˈtrʌɪaŋɡ(ə)l/

noun

plural noun: triangles

1. 1.

   a plane figure with three straight sides and three angles.

   "an equilateral triangle"

Triangles

A triangle has three sides and is made of straight lines. A triangle may be classified by how many of its sides are of equal length. Or, it may be classified by what kind of angles it has.

Types of Triangles by Length

In an equilateral triangle, all three sides are the same length. An equilateral triangle is always equiangular (see below).

In an isosceles triangle, two sides are the same length. An isosceles triangle may be right, obtuse, or acute (see below).

In a scalene triangle, none of the sides are the same length. A scalene triangle may be right, obtuse, or acute (see below).

Types of Triangle by Angle

In an equiangular triangle, all the angles are equal—each one measures 60 degrees. An equiangular triangle is a kind of acute triangle, and is always equilateral.

In a right triangle, one of the angles is a right angle—an angle of 90 degrees. A right triangle may be isosceles or scalene.

In an obtuse triangle, one angle is greater than a right angle—it is more than 90 degrees. An obtuse triangle may be isosceles or scalene.

In an acute triangle, all angles are less than right angles—each one is less than 90 degrees. An acute triangle may be equilateral, isosceles, or scalene.

source:

http://www.factmonster.com/ipka/A0876325.html

Equilateral, Isosceles and Scalene

There are three special names given to triangles that tell how many sides (or angles) are equal.

There can be 3, 2 or no equal sides/angles:

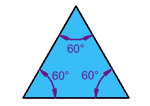	Equilateral Triangle Three equal sides Three equal angles, always 60°
	Isosceles Triangle Two equal sides Two equal angles
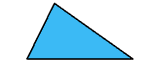	Scalene Triangle No equal sides No equal angles

What Type of Angle?

Triangles can also have names that tell you what type of angle is inside:

	Acute Triangle All angles are less than 90°
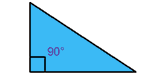	Right Triangle Has a right angle (90°)
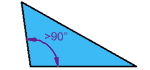	Obtuse Triangle Has an angle more than 90°

Combining the Names

Sometimes a triangle will have two names, for example:

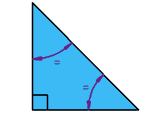Right Isosceles Triangle Has a right angle (90°), and also two equal angles Can you guess what the equal angles are?

source:

https://www.mathsisfun.com/triangle.html

Types of Triangles

Definitions and formulas for triangles including right triangles, equilateral triangles, isosceles triangles, scalene triangles, obtuse triangles and acute triangles
Just scroll down or click on what you want and I'll scroll down for you!
 

right triangle	equilateral triangle	isosceles triangle
obtuse triangle	acute triangle

The right triangle:
	The right triangle has one 90 degree angle and two acute (< 90 degree) angles.  Since the sum of the angles of a triangle is always 180 degrees... y + z = 90 degrees The two sides of the triangle that are by the right angle are called the legs...  and the side opposite of the right angle is called the hypotenuse.

The equilateral triangle:
	In the equilateral triangle, all the sides are the same length (congruent) and all the angles are the same size (congruent).  Since the sum of the angles of a triangle is always 180 degrees, we can figure out the measure of the angles of an equilateral triangle:

The isosceles triangle:
	The isosceles triangle (I can NEVER remember how to spell isosceles) has two sides that are the same length (congruent) and two angles that are the same size (congruent).

 

Popular Topics

• What's a Prime Number?
• Converting Fractions to Decimals
• Order of Operations
• How to Multiply Decimals
• Lines: Finding a Slope With Just Two Points
• The Quadratic Formula
• Solving 2x2 Systems of Equations
• Graphs to Know and Love
• Graphing Functions Easily
• The Unit Circle in Trig

The obtuse triangle:
	Obtuse triangles aren't very smart.  (Look up "obtuse" in the dictionary!) Obtuse triangles have one angle that is greater than 90 degrees.  (Obtuse triangles have one obtuse angle.)

The acute triangle:

Acute triangles are better looking than all the other triangles.  (Pathetic attempt at a math joke.) Acute triangles have NO angles greater than or equal to 90 degrees -- all their angles are less than 90 d egrees.  (Acute triangles have all acute angles.) souce: http://www.coolmath.com/reference/triangles-types\ Triangle properties Vertex The vertex (plural: vertices) is a corner of the triangle. Every triangle has three vertices. Base The base of a triangle can be any one of the three sides, usually the one drawn at the bottom. You can pick any side you like to be the base. Commonly used as a reference side for calculating the area of the triangle. In an isosceles triangle, the base is usually taken to be the unequal side. Altitude The altitude of a triangle is the perpendicular from the base to the opposite vertex. (The base may need to be extended). Since there are three possible bases, there are also three possible altitudes. The three altitudes intersect at a single point, called the orthocenter of the triangle. See Orthocenter of a Triangle. In the figure above, you can see one possible base and its corresponding altitude displayed. Median The median of a triangle is a line from a vertex to the midpoint of the opposite side. The three medians intersect at a single point, called the centroid of the triangle. See Centroid of a Triangle Area See area of the triangle and Heron's formula Perimeter The distance around the triangle. The sum of its sides. See Perimeter of a Triangle Interior angles The three angles on the inside of the triangle at each vertex. See Interior angles of a triangle Exterior angles The angle between a side of a triangle and the extension of an adjacent side. See Exterior angles of a triangle souce: http://www.mathopenref.com/triangle.html